Frosty the Snowman wants to create a small snowman friend for himself. The new snowman needs a base, torso, and a head, all three of which should be spheres. The torso should be no larger than the base and the head should be no larger than the torso.
For building material, Frosty has a spherical snowball with a 6-inch radius. Since Frosty likes to keep things simple, he also wants the radius of each of the three pieces to be a positive integer. Can Frosty accomplish this?
Did you solve the scenario – Can Frosty the Snowman build a new spherical snowman?
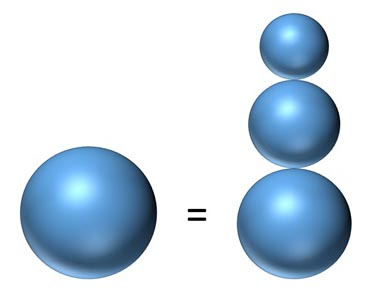
Can you calculate the spherical snowball radii for the new snowman? The original snowball has a 6 inch radius (shown on the far left).
Yes! The formula for the volume of a sphere with radius r is
4/
3 p r
3. Let a, b and c be the radii of the base, torso, and head, respectively, which are integers greater than 0. The problem then amounts to Frosty solving the equation
4/3 p 63 = 4/3 p a3 + 4/3 p b3 + 4/3 p c3
= 4/3 p (a3 + b3 + c3)
By canceling out the factor of 4/3 p from both sides, we're left with
216 = a3 + b3 + c3
As stated in the problem, a>=b>=c. The biggest that a can be is 5, so let's try that first. Subtracting 53 = 125 from both sides gives us
91 = b3+c3
The biggest that b can be is 4, so let's try that next. Subtracting 43 = 64 from both sides gives us
27 =c3
Frosty is in luck, since 33 = 27. Thus we have that 63 = 53 + 43 + 33. Now Frosty can build his new snowman friend to his specifications. In fact, (5, 4, 3) is the only combination of numbers that will work.
Snow Manipulation Solution:
The original snowball has a 6-inch radius. Radii of the new spheres are: 3, 4, and 5.